Exos classiques et autres démonstrations : Différence entre versions
De AgregmathKL
(→Calculer l'exponentielle d'une matrice diagonalisable sans calculer la matrice de passage) |
(→Calculer l'exponentielle d'une matrice diagonalisable sans calculer la matrice de passage) |
||
| Ligne 3 : | Ligne 3 : | ||
Soit <math>A \in \mathcal{M}_n(\mathbb{C})</math>. | Soit <math>A \in \mathcal{M}_n(\mathbb{C})</math>. | ||
soit <math>D=diag(\lambda_1,\dots,\lambda_n)</math> telle que <math>A=Q^{-1}DQ</math>. | soit <math>D=diag(\lambda_1,\dots,\lambda_n)</math> telle que <math>A=Q^{-1}DQ</math>. | ||
| + | |||
Soit <math>P</math> un polynôme tel que <math>P(\lambda_i) = e^{\lambda_i}</math> pour tout <math>i</math>. | Soit <math>P</math> un polynôme tel que <math>P(\lambda_i) = e^{\lambda_i}</math> pour tout <math>i</math>. | ||
| + | |||
Alors <math>P(A) = P(Q^{-1}DQ) = Q^{-1}P(D)Q = Q^{-1}\exp(D)Q = \exp(A)</math> ! | Alors <math>P(A) = P(Q^{-1}DQ) = Q^{-1}P(D)Q = Q^{-1}\exp(D)Q = \exp(A)</math> ! | ||
| + | |||
| + | |||
| + | Ref : perso.univ-rennes1.fr/tristan.vaccon/exponentielle_de_matrices.pdf | ||
Version du 21 juin 2013 à 10:41
Calculer l'exponentielle d'une matrice diagonalisable sans calculer la matrice de passage
Soit 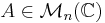 .
soit
.
soit 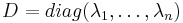 telle que
telle que 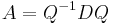 .
.
Soit 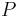 un polynôme tel que
un polynôme tel que 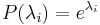 pour tout
pour tout 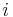 .
.
Alors 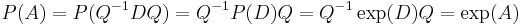 !
!
Ref : perso.univ-rennes1.fr/tristan.vaccon/exponentielle_de_matrices.pdf